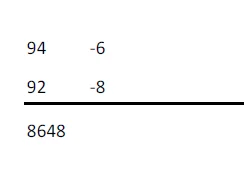Vedic Maths Sutras: What is Nikhilam Navatashcaramam Dashatah?

Nikhilam Navatashcaramam Dashatah is one of 16 Vedic Maths Sutras.

This is a method used to multiply numbers that are closer to the power of 10. The English translation for the same is, All from 9 and last from 10. The powers of 10 from which the difference is calculated are called the Base. These numbers are references to find out whether the number is greater or lesser than the base. The differences in these cases are known as excesses and deficiencies.

To explain the different types of processes in this method we will straightway move to steps and examples so that the Sutra becomes easier to understand.

Nikhilam Type 1

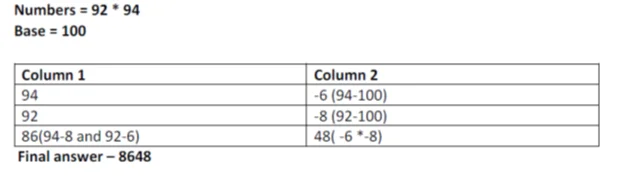
Let us take two numbers, 94 and 92 for multiplication purposes. The Base for both these numbers is 100 as it is the closest.

• Make two separate columns. Write 94*92 in column 1
• Column 2 – 94 is 6 less than 100 and 92 is 8 less than 100.
• Now multiply (-6) * (-8) in column two which gives us 48
• Now we subtract digits diagonally written in column 1 and column 2 i.e we will subtract 8 from 94 and 6 from 92. Both give us the answer 86
• The last step is to append both these answers which give us the final digits as 8648. And here is our answer!

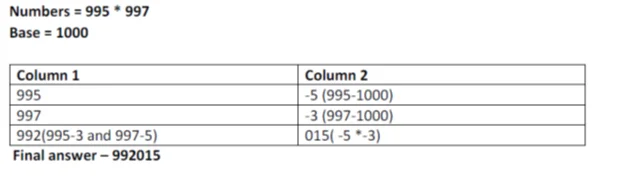
NOW LET US TRY THE SAME METHOD USING 3 DIGIT NUMBERS. We will take 995 and 997. Therefore our base is 1000. Repeating the same steps:

• Make two separate columns. Write 995*997 in column 1
• Column 2: 995 is 5 less than 1000 and 997 is 3 less than 1000.
• Now multiply (-5) * (-3) in column 2 which gives us 15
• Also now that we are multiplying 3 digit numbers the answers even separating must have 3 digits. Therefore add a 0 which answers 015 instead of 15.
• Now we subtract digits diagonally written in column 1 and column 2 i.e we will subtract 3 from 995 and 5 from 997. Both give us the answer 992.
• The last step is to append both these answers which give us the final digits as 992015. And here is our answer!

Nikhilam Type 2

NUMBERS SLIGHTLY GREATER THAN POWER OF 10

Let us take two numbers, 104 and 106 for multiplication purposes. The Base for both these numbers is 100 as it is the closest.

• Make two separate columns. Write 104*106 in column 1
• Column 2 – 104 is 4 more than 100 and 106 is 6 more than 100.
• Now multiply (4) * (6) in column 2 which gives us 24
• Now we add digits diagonally (as they are greater than the base) written in column 1 and column 2 i.e we will add 104 to 6 and 106 to 4. Both give us the answer 110
• The last step is to append both these answers which gives us the final digits as 11024. And here is our answer!

Nikhilam Type 3

NUMBERS CLOSER TO AND PRESENT ON EITHER SIDE OF POWER OF 10.

To learn this method we need to get an idea of one of Vedic Math’s very important processes called the Vinculum process.

Vinculum numbers are those numbers that have 1 negative digit or have a Bar over them (indicating that it is a negative digit).

Through the Place Value System in Vedic Math normal numbers are written as:

2345= 2000+300+40+5

These when they have a bar over them can also be converted into normal numbers. For eg-:

2345= 2000+300-40+5 = 2265

Hence in the above example, the normal number for the above equation is 2265.

Another approach for the same is a process where we start from the right and go to the left. This is a trickier method by is very useful for numbers that have more digits.

Suppose we take the number :

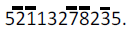
Now to convert this into a normal number, we will follow the below-given steps and move from right to left.

1. Find the 1^st bar digit in the above- given number which in this case is 3 (from the right) and take its 10’s complement.
2. To find the “complement” of a number we subtract the given digit from the number. For eg: The 10’s complement for the number 7 is 3 (10-7)
3. A) If the next digit is a bar digit, take its 9’s complement. Continue until you reach a non-bar digit.

B) Decrement non-bar digit by 1.

• Continue steps 1) and 2) until the whole number is covered.

Therefore, the normal number in the above case will come out to be 47913122175.

COMING BACK TO OUR NIKHILAM PROCESS

NUMBERS CLOSER TO AND PRESENT ON EITHER SIDE OF POWER OF 10.

Let us take two numbers, 94 and 104 for multiplication purposes. The Base for both these numbers is 100 as it is the closest.

• Make two separate columns. Write 94*104 in column 1
• Column 2 – 94 is 6 less than 100 and 104 is 4 more than 100.
• Now multiply (4) * (6) in column 2 which give us 24
• Now we add or subtract digits diagonally (as given) written in column 1 and column 2 i.e we will add 94 and 4 and subtract 6 from 104. Both give us the answer 98
• The last step is to append both these answers which gives us the final digits as 9824. And here is our answer!

This is what we call NIIKHILAM NAVATASHCARAMAM DASHATAH in Vedic Mathematics.

Share with your friends